ETL 1110-2-347
31 May 93
occurrences are commonly observed in the passes
of the Mississippi River and occasionally in other
locations such as the Lake Washington Ship Canal
in Washington and canals in Florida.
7. Densimetric Froude Number
Laboratory experiments have shown that the poten-
tial for a saltwater wedge to form increases for a
densimetric Froude number Fo less than the critical
value Foc = 1 . The densimetric Froude number is
given by
qf
U
Fo
(3)
g′H
g′H 3
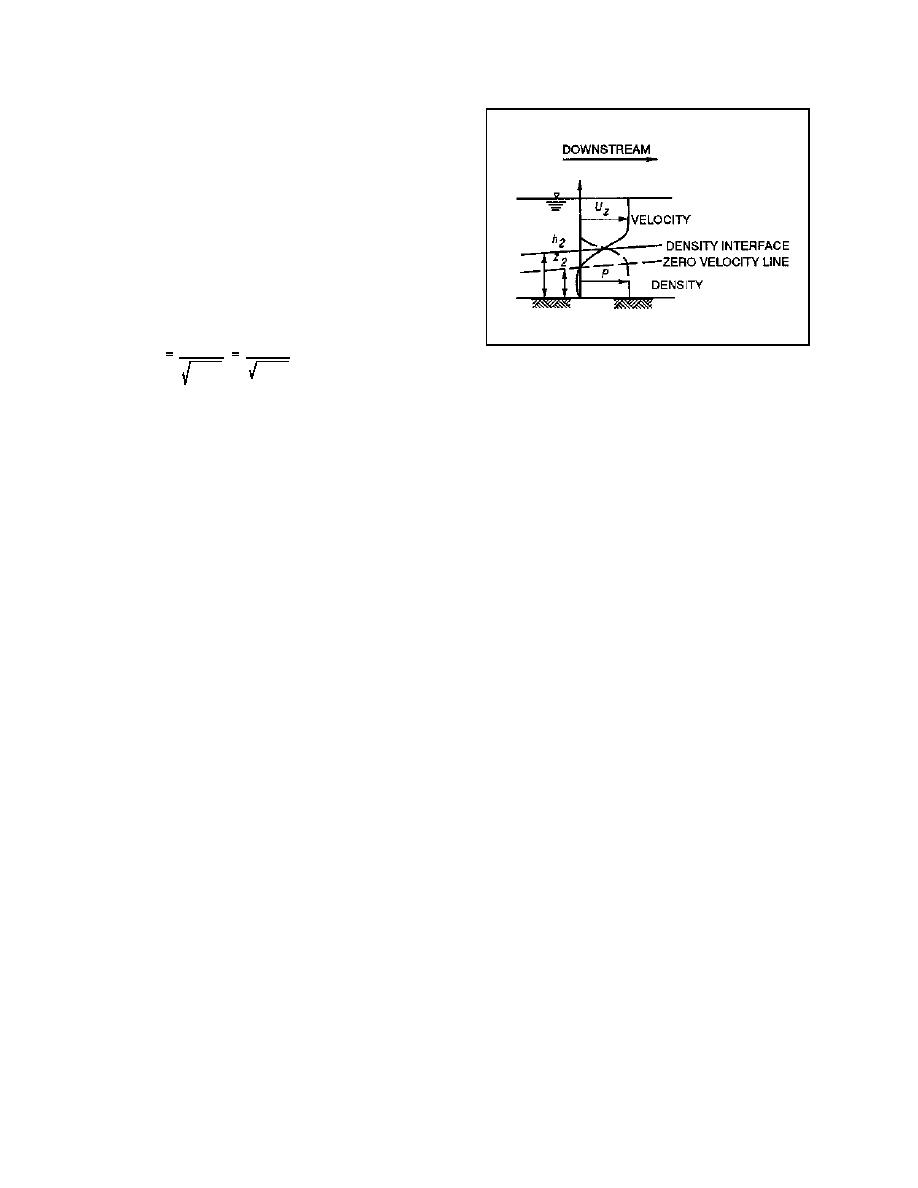
Figure 2. Internal properties of a saline wedge
9. Shape of a Saline Wedge
where
The shape, particularly the height (h2 and z2), of the
saline wedge at a point in the waterway can be
g = acceleration of gravity
determined approximately by the equations of
Keulegan (1966) and Schijf and Schoenfeld (1953),
or more accurately, by laboratory tests and numeri-
cal modeling.
The theoretical critical value is unity; therefore, if
Fo > 1, no saline wedge will form. In practice,
10. Saline Wedge Control
however, values of Fo as low as 0.6 to 0.7 prevent
the formation of a saline wedge. Therefore, for the
The intrusion of the salt wedge can be limited by
condition Fo < 0.6, a salt wedge will form.
control structures. There are three basic types of
control techniques that can conceivably be used:
8. Internal Properties of a Saline Wedge
Venturi control, static control, and dynamic control.
The internal properties of a saline wedge are shown
a. Venturi control. The basic approach with
in Figure 2. There are two distinguishable zones
this option is just as the name implies in that a
within the wedge: the zone or height of the density
short section of the channel is constricted to form a
interface h2 and the height of the zero velocity line
throatlike passage. Therefore, by reducing the local
z2. Velocity and density distributions in the zone
width or depth, the velocities are increased and the
between these two lines are very similar. The
external pressure gradient on the flowing water is
dynamic significance of these zones is that the
reduced. The net result of this type of control is
upper freshwater flow is turbulent, and the motion
that the Froude number at the constriction increases
of the water exerts a high shear stress on the low
so that it exceeds the critical value for limiting salt
turbulence underlying the saltwater layer. This
wedge advancement or preventing its formation.
shear effect tends to destabilize the upper portion of
The drawback to this method is that the constric-
the wedge; however, it is counteracted by the strong
tions must be substantial to produce the desired
density gradient of the saltwater layer. The density
result. Major reductions in depth or increases in
interface is not a distinct line as depicted in the
flow velocity could significantly impede navigation
figure but is mildly active with intermittent transfer
so Venturi controls are often impractical.
and mixing of the salt water into the overlying
freshwater area due to the shear effect of the fresh-
b. Static control. At times it may be neces-
water flow. The lines are not of a constant height
sary to provide a rapidly constructed and temporary
but decrease in height with distance along the
length of the wedge from the ocean landward.
1-2


 Previous Page
Previous Page
