ETL 1110-1-175
30 Jun 97
C (x1, x2) = C (h),
Therefore, when Z(x) has a stationary covariance
function, the variance of Z(x) is constant for all x.
(2-9)
The covariance function can then be standardized
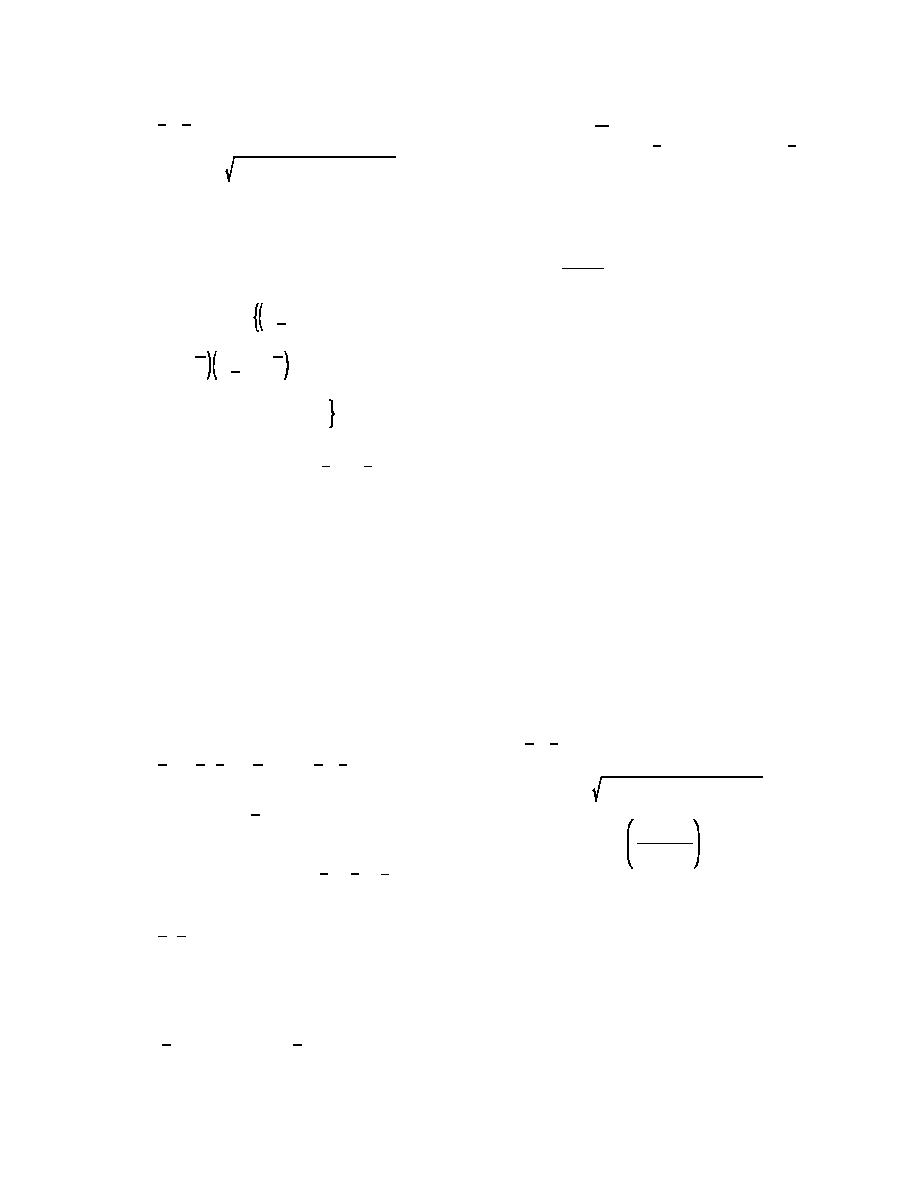
h=
(u1 & u2)2 % (v1 & v2)2
by dividing it by the variance. The resulting
dimensionless function of h is called the spatial
Under this assumption, C(h) can be estimated by
correlation function,
pooling all pairs of observations that are approxi-
mately h units apart and computing a sample
C (h)
D (h) =
(2-14)
covariance function
C (0)
^
C (h) = average Z(x i)
The correlation function is a scale-independent
measure of linear association between values of Z
(2-10)
at different locations. The spatial correlation is
& Z Z(x ) & Z :
j
always between -1 and +1, with a value of zero
indicating no linear association.
h & )h < hij < h % )h
(5) In addition to being stationary, the covari-
where hij is the distance between xi and xj and the
ance function in Equation 2-9 has another import-
average is over all pairs of points such that hij is
ant property. It is also isotropic, or omni-
between h-)h and h+)h. The distance h is called
directional, because it does not depend on the
the lag and )h is called the lag tolerance. There
direction between the two locations. In many
are more effective ways to estimate C(h) other than
HTRW applications, the correlation between
values of Z at two locations is a function of direc-
using Equation 2-10; for example, see Isaaks and
Srivastava (1989). However, because the empha-
tion as well as lag. For example, contaminant
sis in this ETL is on the variogram (to be defined
concentrations in a groundwater flow system might
below) rather than the covariance function, we will
be more highly correlated along a transect in the
not need to use the estimated covariance function.
direction of flow than along a transect perpen-
dicular to the flow. In that case, the covariance
(4) A covariance function is called stationary
function depends on both the lag h and the angle a
if it does not depend on the origin of the coordinate
between locations,
system, that is,
C (x1, x2) = C (h, a),
C (x % b, x % b) = C (x , x )
(2-11)
1
2
1
2
h'
(u1 & u2)2 % (v1 & v2)2, (2-15)
for any given vector, b (Figure 2-1). The covari-
v2 & v1
ance function (Equation 2-9) is stationary because
a ' atan
changing the origin does not change the distance
u2 & u1
between the points. Substituting x1 = x2 = x in
Equation 2-9 yields
Here, a is the angle measured counterclockwise
from the east direction (Figure 2-1). In many geo-
(2-12)
C (x, x) = C (0)
statistical publications or computer packages, the
angle may be defined as clockwise from the north
which, combined with the definitions in Equa-
direction, so care should be taken in defining the
tions 2-6 and 2-8, becomes
appropriate angle in any application. A covariance
function satisfying Equation 2-15 is called aniso-
(2-13)
F2 (x) = C (0) for all x
tropic, or multi-directional.
2-5




 Previous Page
Previous Page
